DiscoverHover Curriculum Guide #13 - How a Hovercraft Turns

DiscoverHover CURRICULUM GUIDE #13
HOW A HOVERCRAFT TURNS
© 2005 World Hovercraft Organization

NAME

DATE

When driving a car, the friction of the rubber tires on the road allows almost instant change in direction when the steering wheel is turned. Piloting a hovercraft, however, is like driving a wet bar of soap, since with hovercraft you slide over most surfaces with little friction. Because ground contact friction is limited, hovercraft are easily affected by wind and surface slope. To maintain directional control of a conventional light hovercraft, two main techniques are employed. The first technique is kinesthetic steering. This occurs when the pilot leans in the direction he or she wants the hovercraft to turn. This increases the drag between the skirt and the ground on that side, causing it to move more slowly than the other side. This causes the hovercraft to turn. Cushion pressure acting on the bottom of the hovercraft hull also facilitates the turn. The second technique uses the rudders of the hovercraft to redirect the thrust air, changing the direction of the air. Remember that momentum is a vector quantity consisting of the product of the mass and the velocity, and that it is conserved in a system where the outside influences are very small compared to the changes within the system. In this case, the total momentum of the hovercraft and of the air must remain constant, so when the hovercraft rudders exert a force on the air to change its direction, the air exerts an equal but opposite force on the rudders according to Newton's Third Law of Motion. This causes a torque on the hovercraft through its approximate center of mass, turning it to face another direction. The table below summarizes quantities in linear and angular motion.

Linear		Angular		Relation
Quantity	Unit	Quantity	Unit
Mass (m)	kilogram [slug] (kg [slug])	Moment of inertia (I)	kilogram meter² [slug foot²] (kg-m² [slug-ft²])	I = m r² (^*)
Position (x)	meter [foot] (m [ft])	Angular position (θ)	radian (rad)	θ = x / (2 × π)
Velocity (v)	meter [foot] per second (m/s [ft/s])	Angular velocity (ω)	radian per second (rad/s)	ω = v × r &omega = Δθ / t
Acceleration (a)	meter [foot] per second per second (m/s² [ft/s²])	Angular acceleration (α)	radian per second per second (rad/s²)	α = a × r α = Δω / t
Momentum (p)	kilogram meter [slug foot] per second (kg-m/s [slug-ft/s])	Angular momentum (L)	kilogram meter² [slug foot²] / second (kg-m²/s [slug-ft²/s])	L = p × r L = I × ω
Force (F)	newton [pound] (N [lb])	Torque (τ)	newton meter [foot pound] (N-m [ft-lb])	τ = F × r F = I × α
^*The moment of inertia given is for a single point. The moment of inertia of an object depends on its shape, its mass, and the distribution of the mass. Also, each axis of rotation has a different moment of inertia.

Table 12-1: Quantities in Linear and Angular Motion

Figure 13-1: Rudders on a Discover Hover One

Example 1:
You are traveling in a straight line on a hovercraft, and the propeller is moving 0.2 m³ [7.06 ft³] of air per second at 90 m/s [295.3 ft/s]. The center of mass of the hovercraft is 1 m [3.28 ft] from the rudders; the hovercraft’s moment of inertia around the center of mass is 95 kg-m² [70.1 slug-ft²]; and the density of the air where you are is 1.22 kg/m³ [2.366×10^-3 slug/ft³]. You then decide to turn left. How far will the hovercraft turn in 3 seconds if the rudders redirect the air at a 40° angle?

Solution:
In order to determine how much the hovercraft turns, we need to find out how fast it rotates. To do that, we find out how much the rudders change the momentum of the air, and therefore how much the hovercraft’s momentum has to change to balance that change. First, we calculate how much air was redirected. We know how fast the air flows in volume, we know how much time we have, and we know how much mass a certain volume of air will have, so we can determine the total mass of the redirected air by finding the volume and then using the density.

Air Volume = Volume Flow Rate × Time
V_air = 0.2 m³/s [7.06 ft³/s] × 3 s
V_air = 0.6 m³/s [21.18 ft³/s]

Air Mass = Volume × Density
M_air = 0.6 m³ [21.18 ft³] × ³1.22 kg/m ³ [2.366×10^-3 slug/ft³]
M_air = 0.732 kg [0.0501 slug]

Now that we know the mass, by using the velocity we can find the momentum of the air when the hovercraft is moving straight.

Momentum = Mass × Velocity
p_{air straight} = 0.732 kg [0.0501 slug] × 90 m/s [295.3 ft/s]
p_{air straight} = 65.88 kg-m/s [14.7 slug-ft/s]

Since momentum is a vector quantity, we note that the momentum we just found is all in the direction straight behind the hovercraft. If we turn the air, some of the momentum will still be straight behind it, but some will be perpendicular to it, either straight to the left or to the right. We find the amounts of each by looking at the angle by which the air is deflected, then we use basic trigonometry to find out how it influences the momentum. Since the thrust system is still running at the same speed, the total airflow is not any different, so we know that the two components of the new momentum have to combine to be the same magnitude as the original.

Diagram of the momentum of the air
Figure 13-2: Components of the momentum of the air.

The component parallel to the hovercraft affects only how fast the hovercraft travels; it does not affect how fast it turns. It will be less than it was when the rudders were straight, so the linear acceleration of the hovercraft will be less than it was before. What interests us now is the perpendicular component, which will tell us how the hovercraft moves.

Perpendicular Momentum = Total Momentum × sin(Angle of deflection)
p_⊥ = 65.88 kg-m/s [14.7 slug-ft/s] sin(40°)
p_⊥ = 42.34 kg-m/s [9.45 slug-ft/s]

This is the horizontal momentum of the air, so it is also the exact opposite of the horizontal momentum on the rudders of the hovercraft. Since the rudders are at the end of the hovercraft rather than in the middle, their change in momentum does not make the hovercraft simply slide sideways; instead, it rotates around its center of mass. To find out how fast the hovercraft turns, we multiply the hovercraft’s momentum by the distance between the rudders and the center of mass (since there is no fixed pivot point, the craft rotates around that point). This gives us the angular momentum. If we divide this number by the moment of inertia of the hovercraft around its center of mass, we get the angular velocity. To find the amount the hovercraft turns, we multiply the angular velocity by the time.

Angular Momentum = Linear Momentum × Radius
L = p × r
L = 42.34 kg-m/s [9.45 slug-ft/s] × 1 m [3.28 ft]
L = 42.34 kg-m²/s [31.0 slug ft²/s]

Angular Velocity = Angular Momentum / Moment of Inertia
ω = L/I
ω = 42.34 kg-m²/s [31.0 slug ft²/s] / 95 kg-m² [70.1 slug-ft²]
ω = 0.446 rad/s

Total Rotation = Angular Velocity × Time
θ = ω × t
θ = 0.446 rad/s × 3 s
θ = 1.338 radians

Radians are handy units for calculations, but it is often easier to understand angles in degrees, so we can convert from radians to degrees by multiplying the angle in radians with the number 180/π

1.338 radians × 180/π = 76.7°

The hovercraft would rotate 76.7°.

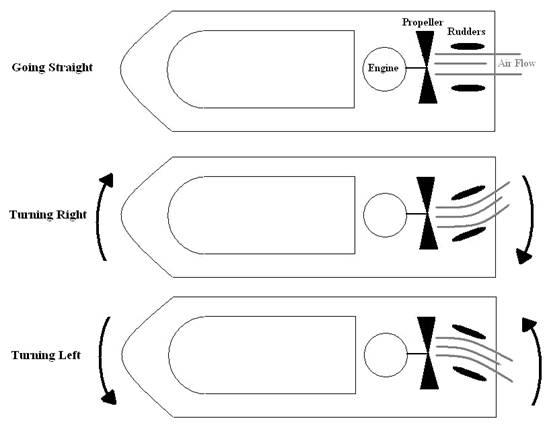
Figure 13-3: Turning of a Hovercraft

You have changed the direction the hovercraft is facing, but the inertia of the hovercraft keeps it traveling in the original direction. Remember Newton’s First Law of Motion? We need a force to change the direction in which the hovercraft is moving. Now that you’re moving nearly sideways, you have a convenient force to do just that – the thrust system of your hovercraft! This is where the other component of the new momentum comes in. It’s really the same principle as the thrust in a straight line, just that now the thrust is not in line with the motion. When a car turns on the road, the tires on the ground experience a lot of friction, which keeps them from moving in a direction other than the direction they are facing. This is why you can steer a car and it will follow the course of the wheels. If the car is driving on ice, however, the coefficient of friction is much smaller, so there is very little friction to bind the car to its course. The same thing happens on a hovercraft, because no component touches the ground with enough force to “grip” the ground. Instead, the hovercraft must provide the force that would have come from friction by pointing the hovercraft into the turn. When this happens, a component of the thrust force is directed toward the center of the turn. A force toward the center of a turn, whether it is caused by the friction from a car's tires, a string keeping a ball from flying off, gravity between the earth and the sun, or the thrust from a turning hovercraft, is called a centripetal force. As you might expect, the centripetal force causes what is called a centripetal acceleration. The object accelerates in the direction of the center of the circle but, since it is also moving in a certain direction, it continually overshoots the center, which is why it moves in a circle (or ellipse). The centripetal acceleration depends on the square of the velocity of the object in the direction perpendicular to the line from the center of the circle to the object, the tangential velocity, and the radius of the circle. The centripetal force is the centripetal acceleration times the mass of the object.

a_c = v_t²/r
Centripetal Acceleration = (Tangential Velocity)² / Radius
F_c = m a_c
F_c = m v²/r

Example 2:
You are in a hovercraft of mass 90 kg [6.17 slugs] operating on hard flat ice on a calm day with air density of 1.204 kg/m³[0.00234 slugs/ft³]at 3.3 m/s [10.8 ft/s]. You are facing east, but the hovercraft is traveling sideways on the ice and its direction of movement is due north. If your propeller moves air at 0.1 m³/s [3.53 ft³/s] and the velocity of the air is 95 m/s [311.7 ft/s], how long will it take to change the hovercraft’s direction of movement to due east, if you keep the angle relative to the direction of travel at 90°? What will be the radius of the turn?

Solution:
For this question, we can use the equation for the centripetal force to get the radius of the turn. To do this, we need the centripetal force, the velocity, and the mass. We know the velocity and mass, so we have to find the force. Since the hovercraft is pointed toward the center of the turn, all of the thrust goes to the centripetal force, so that is what we need to find. For this, we use the form of the thrust equation that works with the mass flow rate and the change in the velocity of the air.

F=[MFR × v]_e−[MFR × v]₀
Thrust Force = (Mass Flow Rate × Velocity) of exiting air − (Mass Flow Rate × Velocity) of entering air

We know the velocity of the exiting air and, since the hovercraft is moving sideways, the air on the intake side of the propeller is moving sideways to it, meaning that the component flowing directly into it is nearly zero. To get the mass flow rate, we simply multiply the volume flow rate by the density of the air.

MFR = VFR × Density
MFR = 0.1 m³/s [3.53 ft³/s] × 1.204 kg/m³[0.00234 slugs/ft³]
MFR = 0.1204 kg/s [8.26×10⁻³ slugs/s]

Now we can plug the values into the thrust equation. The mass flow rate on both sides of the propeller has to be the same, otherwise, the propeller would have to make air magically appear or vanish!

F = (0.1204 kg/s [8.26×10⁻³ slugs/s] × 95 m/s [311.7 ft/s]) − (0.1204 kg/s [8.26×10⁻³ slugs/s] × 0 m/s [0 ft/s])
F = 11.4 N [2.57 lb]

Now that we know the centripetal force, we can find the radius of the turn, since we already know the mass and tangential velocity. We just take the equation for the centripetal force and solve it for the radius, then put in our values (with appropriate unit conversions).

F_c = m v²/r
r = m × v²/F_c
r = 90 kg [6.17 slugs] × (3.3 m/s [10.8 ft/s])²/11.4 N [2.57 lb]
r = 86.0 m [280 ft]

We’re ready now to find how long the hovercraft takes to turn from north to east. To do this, we convert the velocity to angular velocity by dividing it by the radius. Once we have this, we know that the angle it travels is the angular velocity multiplied by the time. We know the angle is 90°, which is equal to π/2 radians, and will have just found the angular velocity, so we can use those numbers to find the time.

ω = v_t/r
ω = 3.3 m/s [10.8 ft/s]/86.0 m [280 ft]
ω = 0.0384 rad/s
t = θ/ω
t = (π/2)rad/(0.0438 rad/s)
t = 35.9 s

Conclusion:

The hovercraft would take 35.9 seconds to turn from north to south, and the radius of the turn would be 86.0 m [280 ft].

The more thrust air you can redirect, the faster the hovercraft will respond to directional changes. This is why hovercraft usually have at least 2 rudders. Some models may have a cascade of as many as perhaps 5 rudder blades to change the direction of nearly all the thrust air. To be effective at low speeds, rudders must also turn at least 60 degrees in each direction. Rudders are usually shaped like a symmetrical airfoil to minimize drag (air resistance) and to maintain smooth airflow as the angle increases in order to prevent the rudder blade from stalling.

Quiz Questions.

Can we use horizontal rudders instead of vertical ones? Why or why not?
What will happen if the rudders are rotated 90˚?

©2005 World Hovercraft Organization
All rights reserved. Copies of this Curriculum Guide may be printed for classroom use exclusively by DiscoverHover registered members. This Curriculum Guide and all materials contained in the DiscoverHover web site are protected by copyright laws and may not be reproduced, republished, distributed, or displayed on any other web site without the express prior written permission of the World Hovercraft Organization.